Las matemáticas prueban que los vampiros no existen

He encontrado en Collision Detection esta curiosa prueba de que los vampiros no existen, si lo hiciesen no habría humanos en tan sólo 30 meses, y eso con una suposición bastante simplista...

Math proves that the Buffy universe harbors no more than 512 vampires

 

 

 

 

 

 

 

 

One of the most totally fun areas of publishing in recent years has been the emergence of books that probe the scientific bases of fictional universes -- like The Physics of Star Tre or The Science of Star Wars. Thus I was tickled to discover a paper called "Ghosts, Vampires and Goblins: Cinema Fiction vs. Physics Reality", authored by Costas Efthimiou and Sohang Gandi (PDF here). Among their conclusions? Vampires can't exist.

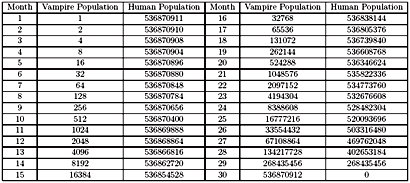
Why? Because they'd quickly depopulate the earth. To prove it, the scientists do some calculations by picking a random year in history -- 1600, specifically -- and imagining what would happen if one person suddenly appeared on earth. They assume, for the sake of argument, that a vampire needs to feed "only once a month", and that in the course of feeding, the vampire turns its victim into another vampire. They note that the global population of humans was 536,870,911 in the year 1600.

Then the calculations begin. If a single vampire fed on a single human in the first month, this would create two vampires -- and decrease the human population by one, leaving it at 536,870,911 - 1 = 536,870,910. In the second month, those two vampires would each feed, transforming two people into vampires -- so you get four vampires and a human population of 536,870,911 - 3 = 536,870,908. So you can see where this is headed. The vampire population is increasing in a geometric progression, and the population of humans is similarly decreasing -- and at that rate, the authors calculate, the entire human population would be transformed into vampires in only 30 months. QED!

Sure, humans could increase their numbers by having children -- but the birth rate could never keep pace. Thus, the authors' implacable logic leads them to only one conclusion:

We conclude that vampires cannot exist, since their existence contradicts the existence of human beings. Incidently, thelogical proof that we just presented is of a type known as reductio ad absurdum, that is, reduction to the absurd. Another philosophical principal related to our argument is the truism given the elaborate title, the anthropic principle. This states that if something is necessary for human existence, then it must be true since we do exist. In the present case, the nonexistence of vampires is necessary for human existence. Apparently, whomever devised the vampire legend had failed his college algebra and philosophy courses.

It's worth checking out the full paper, if only to see a bigger version of the their spreadsheet (excerpted above) showing how the vampire-vs-human population evolves, month-by-month.

This would seem to strike a horrible blow to the whole concept of Buffy the Vampire Slayer, eh? And indeed, when this study came out last year, Buffy fans worldwide wept hot, bitter tears.

But wait! The whole point behind the Buffy universe is that there's a slayer out there killing vampires and keeping their population down. This is something the authors didn't consider in their paper. So couldn't a vampire killer simply slaughter vampires as fast as they're created?

Sure -- except then the math gets even more interesting.

Because the thing about the Buffy universe is that the population of vampires is reasonably stable. There are a fair number of vampires around, but not enough to overwhelm the earth. But as it turns out, if you look at that chart above, there's a very narrow vampire-population window at which equilibrium can be kept.

That's because powers of two increase slowly at first, then at a hellacious rate. Think of it this way: According to the numbers calculated by the academics, at month five in the year 1600, there are only 16 vampires. That's such a paltry number than any self-respecting slayer could quickly dispatch them in a few evenings, and the vampire menace would permanently be extinguished. But at month 12 -- only a few months later -- the number of vampires, unchecked, rises to 2,048. That's probably too many vampires for a slayer to squelch in a single month.

So the really sweet spot seems to be months eight to ten -- when the vampire population would range from 128 to 512, respectively. Those seem like realistic numbers of vampires for a slayer to kill in a single month, assuming she kills 2 to 8 per night. With that kill-ratio, a slayer each month could kill enough vampires to knock the population back a month or two. This would keep the vampire menace sustainable -- neither fully depleting it nor letting it race out of control.

So there you go. I've calculated the precise number of vampires that probably exist in a Buffy universe: No more than 512. Granted, this number could change depending on one's assumptions of how many vampires a single slayer can kill in a month, or how many slayers exist at any one point in time, or how many other people might be killing vampires in addition to the slayer(s). I'm not actually a Buffy fan, so I'd be interested to hear what other assumptions that more-informed fans might make -- and calculations would ensue.